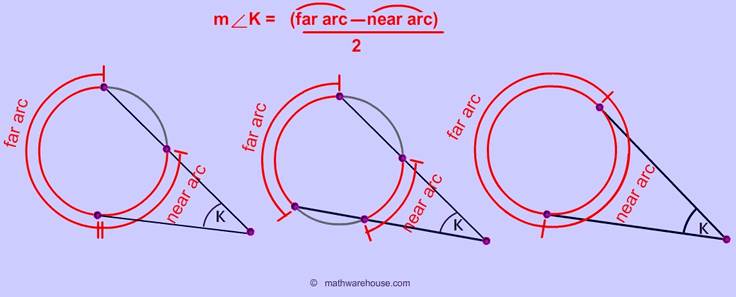
Far Arc near arc formula" />The three theorems for the intercepted arcs to the angle of two tangents, two secants or 1 tangent and 1 secant are summarized by the pictures below. If you look at each theorem, you really only need to remember ONE formula.
The angle formed by the intersection of 2 tangents, 2 secants or 1 tangent and 1 secant outside the circle equals half the difference of the intercepted arcs! Therefore to find this angle (angle K in the examples below), all that you have to do is take the far intercepted arc and near the smaller intercepted arc and then divide that number by two! That's why we call this the Far Arc Near Arc theorem (sometimes abbreviated Farc - Narc) .
All of the formulas on this page can be thought of in terms of a "far arc" and a "near arc". The angle formed outside of the circle is always equal to the the far arc minus the near arc divided by 2.
Far Arc near arc formula" />
Look up above to see the easy way to remember the formulas
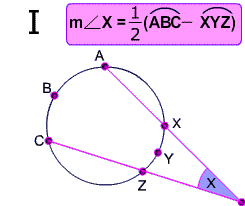
The measure of an angle formed by a secant and a tangent drawn from a point outside the circle is $$ \frac 1 2 $$ the difference of the intercepted arcs .
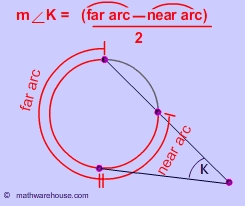
Remember that this theorem only used the intercepted arcs . Therefore, the red arc in the picture below is not used in this formula.
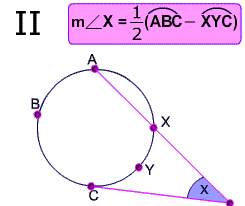
The measure of an angle formed by a 2 secants drawn from a point outside the circle is half the the difference of the intercepted arcs:
In the picture below, the measure of $$ \angle x$$ is $$ \frac 1 2 $$ the difference of the arcs intercepted by the two secants.
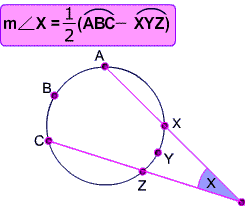
Remember that this theorem only makes use of the intercepted arcs. Therefore, the red arcs in the picture below are not used in this theorem's formula.
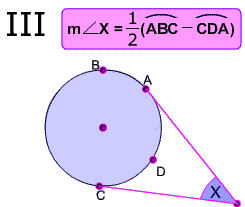
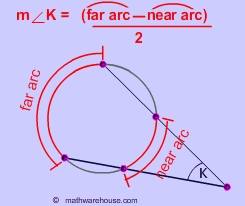
The measure of an angle formed by a two tangents drawn from a point outside the circle is $$\frac 1 2 $$ the the difference of the intercepted arcs .
In one way, this case seems to differ from the others-- because all circle is included in the intercepted arcs. Since both of the lines are tangents, they touch the circle in one point and therefore they do not 'cut off' any parts of the circle.
